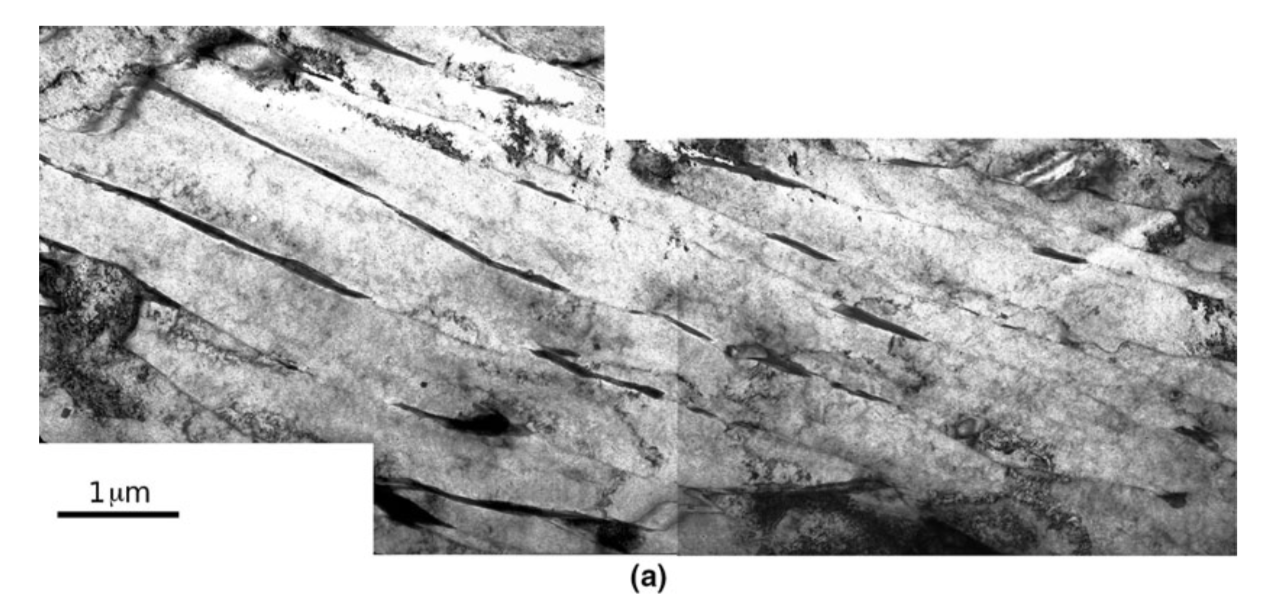
 | Transmission electron micrograph of X80 steel after isothermal transformation at 600oC for 30 s. Shows clear plates of bainitic ferrite. |
When ferrite grains in iron nucleate at different locations and then grow unimpeded. However, they will eventually touch each other, a phenomenon known as hard impingement. Obviously, they cannot grow through each other so they grow in directions that eventually allow the grains to fill all space. Their shape then does not reflect the morphology during unimpeded growth.
Here is an example from X80 pipeline steel, which has a chemical composition given approximately by Fe-0.05C-1.6Mn-0.1Nb\,wt%. The carbon concentration is close to the solubility in ferrite, so during continuous cooling, all the austenite is consumed.
Transformation for a very short time of 30 s at 600oC shows beautiful plates of bainite in a transmission electron microscope. However, allowing it to complete means that the plates have to fill all space and therefore show an irregular appearance following impingement.
More details in associated publication.
| Transmission electron micrograph of X80 steel after isothermal transformation at 600oC for 30 s. Shows clear plates of bainitic ferrite. |
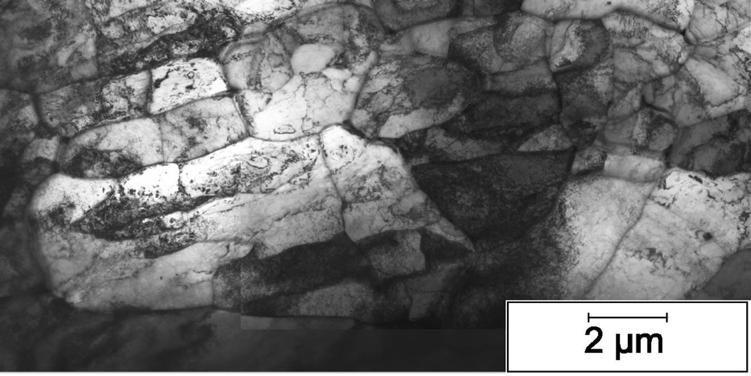 | Transmission electron micrograph of X80 steel after completion of transformation following production. The plates of bainite have impinged and fill all space, therefore appearing irregular when compared with the neat shape of bainite. The structure therefore can confuse because this is not the shape from unrestricted growth. |
This experiment was done to illustrate the fact the shape of particles during unimpeded growth is different from that expected when the touch and fill all space. Please conduct a risk assessment before attempting the task.
225 g rolled oats, 120 g flour, 5 g baking powder, 3 g teaspoon baking soda, 200 g salted butter, 250 g granulated sugar, 1 medium egg (not essential, can be replaced by ripe bananna).
The equipment is a large mixing bowl, a baking tray, grease-proof paper and an oven.
 | The raw cookies laid out as if they have nucleated homogeneously but at different locations so that unimpeded lateral growth can occur. Note that the mixture flows at high temperature, which drives the lateral growth accompanied by vertical shrinkage. The driving force is gravity. |
 | Lateral growth eventually leads, during the 13 min and 5s heat treatment, to impingement with a balancing of forces between the cookies. This creates grain boundaries and triple junctions, a polycookie. The shape is no longer a reflection of what happened during unimpeded growth. |
 | After eating a few cookies (note risk assessment), it is clear that the boundaries are weak interfaces, and that the triple junction particularly weak. There is clearly a greater free volume at these defects in the polycookie so diffusion or even the flow of air should be much easier from the top to bottom surfaces, or laterally. |
The first scientist, according to Natalie Hayes ("comedian talks classics", BBC), was Aristotle, whose evidence based logic, the willingness to admit incorrect hypotheses, et cetra set the scene for the scientific method. After Plato's school he went to Lesbos and created biology as we know it now. His repeated observations were a key feature of his method.
Here Jean Pomfrett (who assembled a Stirling engine) now turned her hand, with the help of her grandson, to repeating the experiments described above, with some astonishing results.
The work might have answered in part, one of the suggestions made above for future work. However, further work is need to understand why the flow in her experiments was so dramatic. (nota bene: a proper piece of work finishes with unanswered queries - or as Derek Fray would say, scientists like to travel but never to reach their destination.)
 The raw cookies prior to heat treatment |
 The flow during heat treatment was quite remarkable, the raw cookies appearing to coalesce into a "single crystal" analogue, with only minute vestiges of the original form visible. Those might be the analogue of "low misorientation boundaries because they could not be separated without much work (free energy)". Obviously, this object is too large for one person to eat. What is to be done? |
 A machining operation creates the form expected but in modern jargon, instead of additive manufacturing this involves subractive manufacturing. Wonder if there is anything such as multiplicative or divsive manufacturing? |
Stirling engine, by Jean Pomfrett |
In continuing experiments, Mathew Peet substituted the egg by a ripe banana.
This seems to have reduced the flow during heat treatment. However, Mathew points out that because of the numerous experiments, he had to limit the number of cookies made and eaten. Therefore, the ingredients were halved in quantity and the raw forms were smaller.
Furthermore, olive oil was used on grease proof paper rather than butter to enhance the vegan-ness of the recipe.
The heat treatment was interrupted for inspection to characterise the intermediate stage of the process, by removing from oven for around 30 seconds.
In summary, given that there are many variables, we propose that with the steady accumulation of data, this would be an ideal project for machine learning.
 The raw blanks. |
 After 10 min 23 s |
 After 10 min 23 s |
 |
 |
The units of cookie radius are arbitrary, with each cookie-size calibrated by the tray size. It appears as though the strict 3r3 - 3ro3∝Vt, where V is assumed to be proportional to ro2 is not obeyed because the size does not change after 623 s (may even show some shrinkage, perhaps evaporation of soft matter?). The initial gradient is linear (!) as expected, but then flattens out; there presumably is a limit beyond which the driving force for spreading is not sufficient. Could do with more points in the initial stages, but note that there is unexplained scatter. |
| PT Group Home | Materials Algorithms |
